Solid Klein bottle

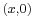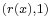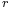In mathematics, a solid Klein bottle is a 3-manifold (with boundary) homeomorphic to the quotient space obtained by gluing the top of $\scriptstyle D^2 \times I$ (cylinder) to the bottom by a reflection, i.e. the point $\scriptstyle (x,0)\,$ is identified with $\scriptstyle (r(x), 1)\,$ where $\scriptstyle r\,$ is reflection of the disc 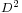$\scriptstyle D^2\,$ across a diameter.

Note the boundary of the solid Klein bottle is a Klein bottle.

Alternatively, one can visualize the solid Klein bottle as the trivial product 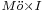$\scriptstyle M\ddot{o}\times I$ , of the möbius strip and an interval $\scriptstyle I=[0,1]$ . In this model one can see that the core central curve at 1/2 has a regular neighborhood which is again a trivial cartesian product: $\scriptstyle M\ddot{o}\times[\frac{1}{2}-\varepsilon,\frac{1}{2}%2B\varepsilon]$ and whose boundary is a Klein bottle as previously noticed